
Visiting again the rectangular tube (Figure 3), if the global axis is a centroidal one (passing through middle height of the section), then using method 'b', the centroids of the two rectangular subareas, are located on middle height too, and therefore feature zero distance from the global axis. Simpler subareas, generally require less calculations to find their centroid, but also the position of subarea, relative to the global axis is important. The work we have to do in this step, depends on the way the subareas have been defined, in the first step. With step 2, we determine the centroid of each subarea, specifically its distance from the global axis.
Method 'b' utilizes a negative subarea (cut-out) Two alternative ways to define subareas for the rectangular tube (top-left). Apart from this, all other steps of the calculation procedure are normally applicable for the negative subarea. Following this pattern, the moment of inertia of the negative subarea is going to be subtracted from the respective one of the outer perimeter, in order to find the resulting moment of inertia of the rectangular tube.
The latter, if subtracted from the former, produces the composite shape and for this reason is characterized as negative subarea. But a more efficient method is to define just two rectangular subareas, one for the outer perimeter and one for the inner perimeter (method 'b'). Conventionally, it can be decomposed to 4 rectangular subareas, one for each of the walls, that added together produce the composite shape (method 'a'). Take as an example the rectangular tube in the next figure. Sometimes, it is more efficient to define negative subareas, that when subtracted from another, bigger subarea, produce the total composite shape. In particular, method 'b' seems a bit more efficient, since it employs one less subarea, compared to method 'c'. For each one of their subareas, there are easily available analytical formulas, we can use to calculate their moments of inertia around a parallel to global axis. Methods 'b' and 'c' on the other hand, do not present such a problem. Surely, it can be done this way, but it is not very efficient calculation wise. Firstly, we have to find the moments of inertia I x and I y around the inclined centroidal axes of the rectangle, and then transform them around a horizontal axis, using the formulas for axis rotation. No formula is available for this particular angle of inclination of the rectangle that gives its moment of inertia around an axis parallel to the global one. Method 'a' seems more simple, and employs the least number of subareas, but the calculation of the moment of inertia of the central inclined rectangle is rather hard. Three alternative ways to divide the top-left gray area to subareas, in order to find its moment of inertia around a horizontal axis. With method 'a', the number of subareas is 4, with method 'b' it is 5 and and with method 'c', 6. The global axis of rotation is indicated with red dashed line.

The following picture demonstrates a case of a composite area, that is decomposed to smaller subareas, using three different methods (among many others possible). Efficiency is important, because sometimes there are many ways to decompose an area, but not all of them are equally easy for calculations. While doing so, we must ensure that we can efficiently obtain the moment inertia of each subarea, around a parallel axis. With step 1 we aim to divide the complex area under investigation to smaller and more manageable subareas. Flowchart for the calculation of the moment of inertia of a composite area Add (or subtract for negative subareas, see examples) the moments of inertia from the last step.įigure 1.Apply the Parallel Axes Theorem to find the moment of inertia of each subarea around the global axis.Determine the moment of inertia of each subarea, around a parallel axis, passing through subarea centroid.Determine the distance from global axis of the centroid of each one of the subareas.Identify simply shaped subareas the composite area can be decomposed to.In general, the steps for the calculation of the moment of inertia of a composite area, around an axis (called global axis hereafter), are summarized to the following:
COMPOSITE MOMENT OF INERTIA CALCULATOR HOW TO
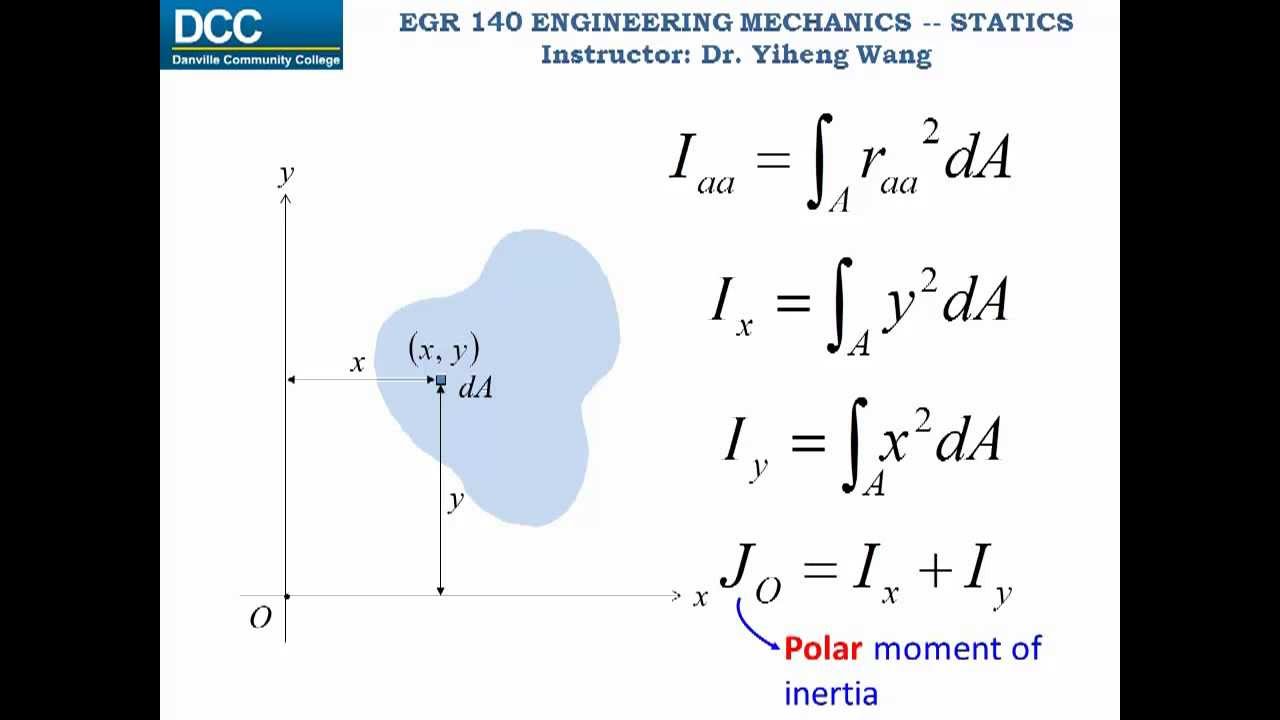
In this article, it is demonstrated how to calculate the moment of inertia of complex shapes, using the Parallel Axes Theorem. The possible shape geometries one may encounter however, are unlimited, but most of the times, these complex areas can be decomposed to more simple subareas. The given analytical formulas for the calculation of moments of inertia usually cover, just a handful of rather simple cases.


 0 kommentar(er)
0 kommentar(er)
